Vectors
Notation
The set of all real numbers is denoted as $\mathbb{R}$ and the set of all vectors with $N$ coordinates, whose entries are real numbers, is denoted by $\mathbb{R}^N$.
Vectors are written as by $\boldsymbol{v}$ or $\vec{v}$.
The vector whose every entry is zero is called a zero vector.
But note that if $\boldsymbol{v} = \boldsymbol{0} \in \mathbb{R}^2$ and $\boldsymbol{u} = \boldsymbol{0} \in \mathbb{R}^3$, then $\boldsymbol{v} \neq \boldsymbol{u}$, nor can arithmetic operations, such as addition or subtraction be performed.
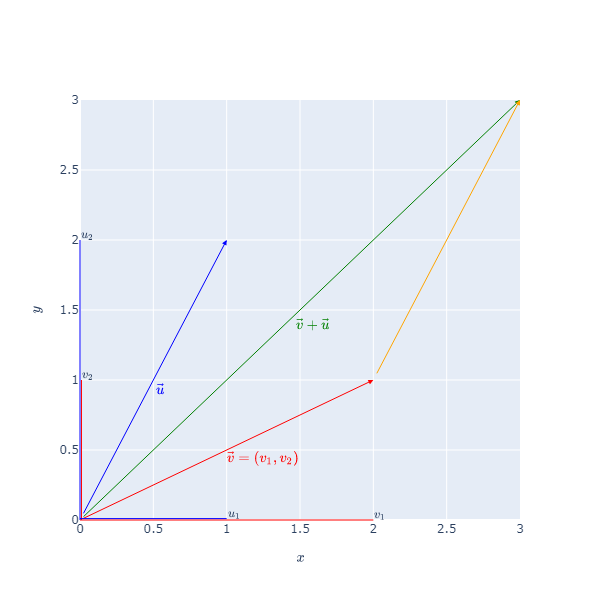
Given some $k$ vectors $\vec{v}_1, \vec{v}_2, \ldots, \vec{v}_k \in \mathbb{R}^{n}$, then their linear combination is an expression of the form
$$ \begin{equation*} a_1 \vec{v}_1 + a_2 \vec{v}_2 + \ldots + a_k \vec{v}_k \end{equation*} $$where $a_1, a_2, \ldots, a_k \in \mathbb{R}$, i.e. are scalars.
For two vectors $\boldsymbol{a}$, $\boldsymbol{b} \in \mathbb{R}^{n}$, where $\boldsymbol{a}= \left(a_1, a_2, \ldots, a_n \right)$, the dot product of the two vectors is given by
$$ \begin{equation*} \boldsymbol{a} \cdot \boldsymbol{b} = a_1 b_1 + a_2 b_2 + \ldots a_n b_n. \end{equation*} $$The result is a scalar.
The dot product can be generalised for more general vectors, called an inner product, written as $\langle \cdot, \cdot \rangle=c$, where $c$ is scalar.
The length of a vector is denoted by $\left| \vec{v} \right| = \sqrt{\vec{v} \cdot \vec{v}}$.
A unit vector has length one. A non-zero vector can be transformed into a unit vector by the transformation $\vec{v} \mapsto \vec{v} / \left| \vec{v} \right|$. This process is often called normalization, as the norm of a vector is often defined as $\sqrt{\langle \vec{v}, \vec{v} \rangle}$.
One can show that, for vectors $\boldsymbol{a}, \boldsymbol{b} \in\mathbb{R}^2$, then
$$ \begin{equation*} \boldsymbol{a} \cdot \boldsymbol{b} = \left| \boldsymbol{a} \right| \left| \boldsymbol{b} \right| \cos\theta. \end{equation*} $$Thus, any two vectors, $\vec{v}$ and $\vec{u}$ are said to be orthogonal (or perpendicular) if $\vec{v} \cdot \vec{u}=0$.
Thus, the angle between two vectors is given by
$$ \begin{equation*} \cos\theta = \dfrac{\boldsymbol{a} \cdot \boldsymbol{b}}{\left| \boldsymbol{a} \right| \left| \boldsymbol{b} \right|}. \end{equation*} $$The Cauchy-Schwarz inequality states that the magnitude of the between two vectors is always less than or equal to the product of the magnitudes of the two vectors, i.e.
$$ \left| \vec{v} \cdot \vec{w} \right| \leq \left| \vec{v} \right| \left| \vec{w} \right|. $$The triangle inequality states that the sum of the lengths of any two sides must be greater than or equal to the length of the remaining side. Formally, this is expressed as
$$ \left| \vec{v} + \vec{w} \right| \leq \left| \vec{v} \right| + \left| \vec{w} \right|. $$It can be derived from the Cauchy-Schwarz inequality.
For the Cauchy-Schwarz inequality, when the vectors $\vec{v}$ and $\vec{w}$ lie on the same line, then $\left| \vec{v} \cdot \vec{w} \right| = \left| \vec{v} \right| \left| \vec{w} \right|$.
For the triangle inequality, when the vectors point in the same direction, then the $\left| \vec{v} + \vec{w} \right| = \left| \vec{v} \right| + \left| \vec{w} \right|$.