Approximations for acoustically excited bubble cluster dynamics
 Bubble radius
Bubble radius
Abstract
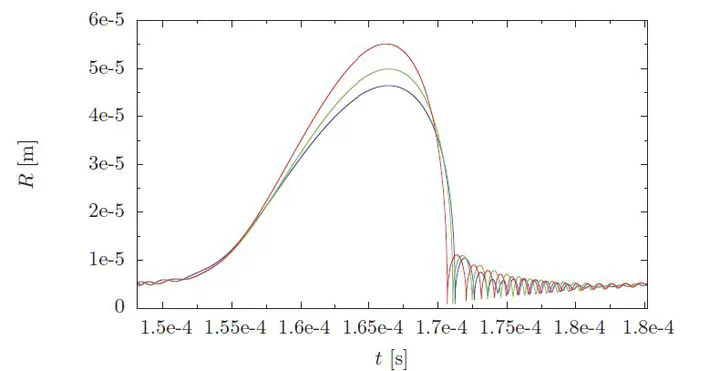
In this paper the effect of interaction on the expansion of a bubble in a regular monodisperse cluster is investigated. By a geometric construction a two-dimensional ordinary differential equation with an exact expression for first-order bubble interactions is derived for an n-bubble model. An approximate equation is derived for the rapid expansion of the bubble which can be solved yielding an analytic expression for the collapse of a bubble which undergoes inertial cavitation. It is then demonstrated that the maximum volume of a bubble in a cluster is considerably less than that of a single bubble. This result is of significance as typically the dispersion relationship, the wave speed and the co-efficient of attenuation are calculated using a single bubble model and summed for the total number of bubbles to yield the void fraction. Furthermore it is shown that the maximum radius of a bubble in the cluster is considerably smaller than that of a single bubble, yet the duration of the collapse phase is only weakly dependent on the number of bubbles. Hence, it is conjectured that the likelihood of fragmentation due to Rayleigh–Taylor instability is reduced. The results from the analysis are in good agreement with full numerical simulations of multi-bubble dynamics, as well as experimental observations
Type
Publication
Journal of Physics: Conference Series